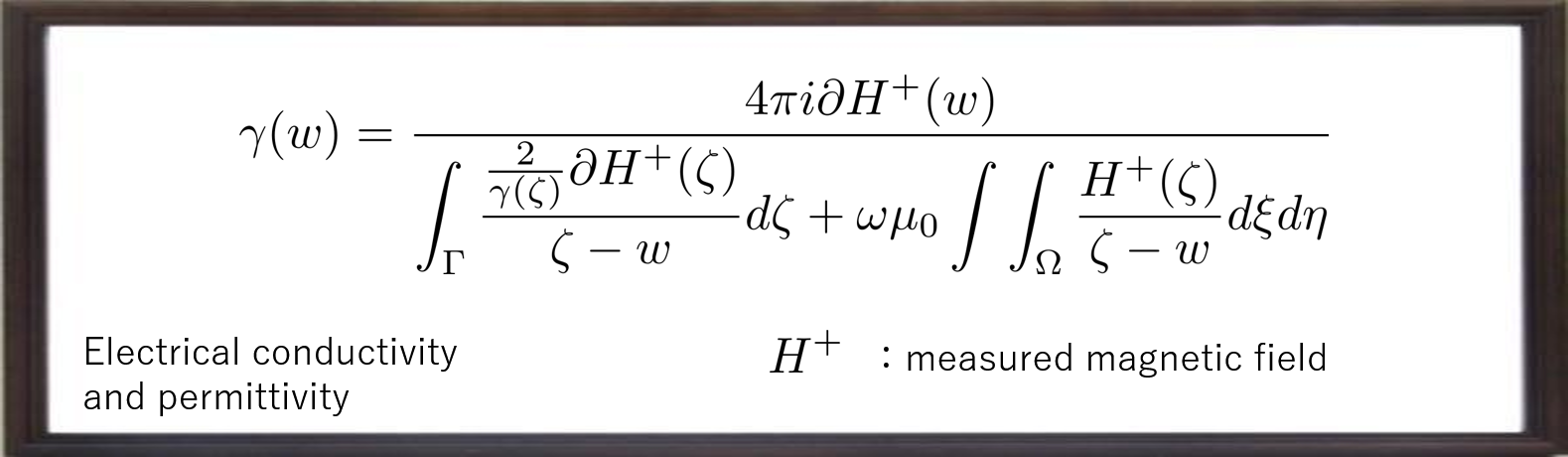Takaaki NARA: Research Theme
The interview was published on
the SECOM Science and Technology Foundation's website.(Japanese only)
Direct methods for inverse problems
We have developed mathematical theories for inverse problems that can explicitly
reconstruct the cause of a phenomenon in terms of measured data.
Conventional nonlinear-optimization-based methods for inverse problems are generally
disadvantageous in that the algorithms often converge to a local minimum, that is,
a false solution, if an appropriate initial estimate is not given. In contrast,
the direct method can estimate a solution without iterative computing of the forward solution.
These methods completely clarify the mechanisms for how the law of causality is inverted,
and hence provide solid theoretical foundations for inverse problems.
In addition, they can provide appropriate initial estimates for use in conventional iterative methods,
which is normally a challenging problem, and thus are highly useful in many practical situations.
Furthermore, they can be used for evaluating the stability of inverse problems.
The main topics of the research are as follows:
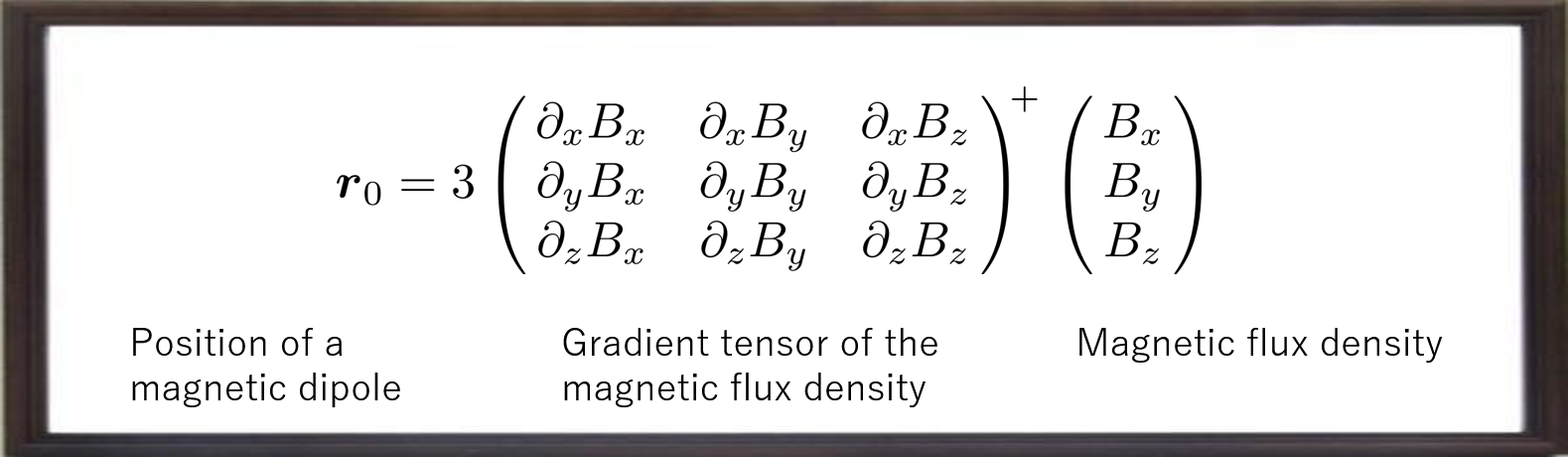
Localization of a magnetic dipole: Many inverse problems can be formulated as an
inverse dipole source problem. For example, in the non-destructive inspection of ferromagnetic pipes,
the position of a crack can be estimated as that of an equivalent magnetic dipole. For this problem,
we have derived a very simple, direct reconstruction formula that expresses the position of a dipole,
irrespective of its moment, in terms of the magnetic field and its gradient tensor.
Applications utilizing this method can allow for a magnetic field source to be located with only a very compact sensor.
See the following papers for more details:
T.Nara, S.Suzuki, and S.Ando: A closed-form formula for magnetic dipole localization by measurement of its magnetic field and spatial gradients, IEEE Transactions on Magnetics, Vol. 42, No. 10, pp. 3291-3293, 2006.
T.Nara and W.Ito: Moore-Penrose generalized inverse of the gradient tensor in Euler's equation for locating a magnetic dipole, Journal of Applied Physics, vol. 115, 17E504, 2014.
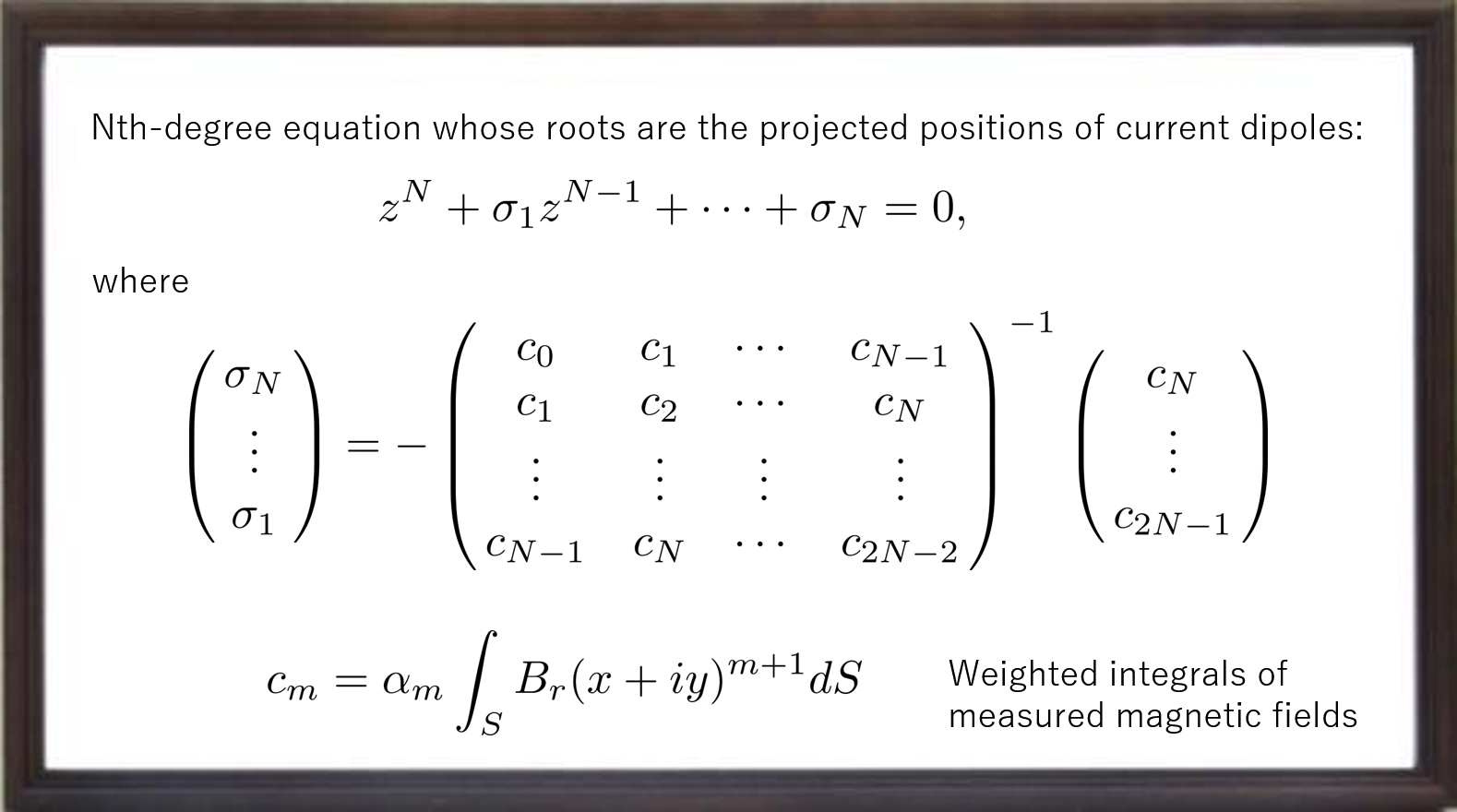
Localization of multiple current dipoles: We have derived a method to identify the
locations of multiple current dipoles as the roots of an algebraic equation determined by
measurements of their magnetic field. Using the characteristics of a Hankel matrix
composed of the weighted integral of measured data, the number of the current dipoles
is also estimated. This method can be applied to biomagnetic inverse problems.
See the following paper for more details:
T.Nara, J.Oohama, M.Hashimoto, T.Takeda and S.Ando: Direct reconstruction algorithm of current dipoles for vector magnetoencephalography and electroencephalography, Physics in Medicine and Biology, Vol. 52, pp. 3859-3879, 2007.
Reconstruction of electrical impedance of materials and structures: We have
derived an explicit reconstruction formula for the electrical conductivity and
permittivity inside a material or a structure in terms of the generated magnetic field
inside it. It is based on the generalized Cauchy formula, a beautiful and powerful
integral formula in complex analysis. This formula can be used for the electrical
properties tomography inside the human body.
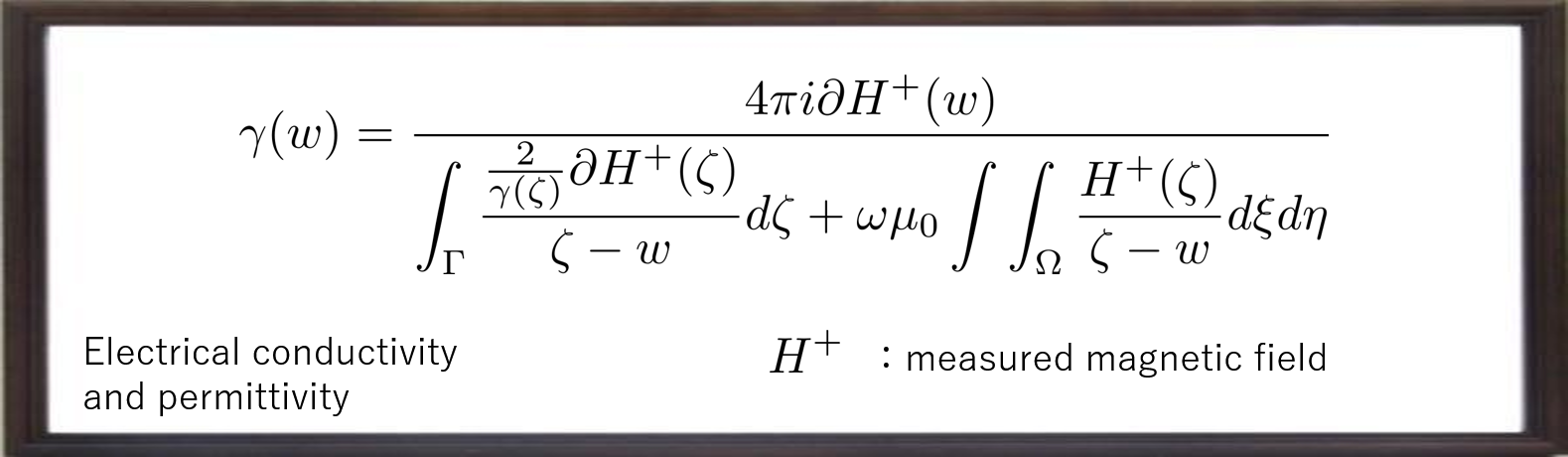
See the following paper for more details:
T. Nara, T. Furuichi and M. Fushimi, An explicit reconstruction method for magnetic resonance electrical property tomography based on the generalized Cauchy formula, Inverse Problems, vol. 33, 105005, 2017.
Magnetoencephalography inverse problem: application to identification of epileptic foci
Magnetoencephalography (MEG) and Electroencephalography (EEG) inverse problems
are bio-electromagnetic inverse problems in which the neural current sources inside a
human brain are estimated from measurements of the magnetic field and the electrical
potential on the surface of the head. Important applications include localization of an
epileptic focus using non-invasive measurements. Although conventionally, a single
equivalent current dipole source can be estimated in clinical practice, those methods can
only identify the center of an epileptic focus and not its spatial extent. For improved
surgical outcomes, identifying the shape and spatial extent of the focus would provide valuable information.
We can formulate an inverse problem to identify the shape of the domain inside which
the neural current flows on the very complex and convoluted brain surface. By using a
mapping from the brain surface to a sphere, we have proposed a novel algorithm to
identify the shape of the source domain. This research has been conducted in
collaboration with the Department of Medicine at Hiroshima University and the University of Tokyo Hospital.
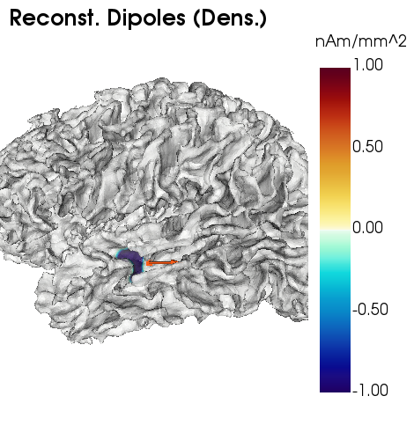
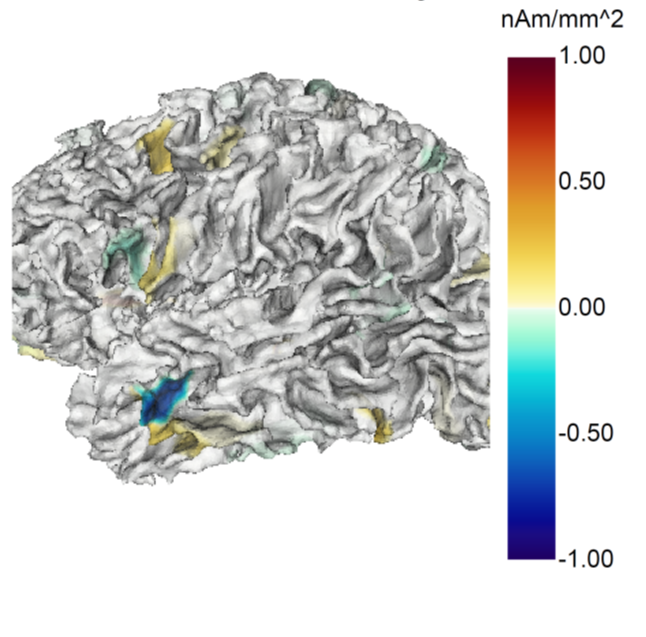
(left) A conventional method assuming an equivalent current dipole can estimate only the location of the center of an epileptic focus (red arrow), whereas the proposed method can identify the shape of the domain (blue).
(right) Results using a conventional imaging approach with L1-norm and total variation regularization: the estimated current sources are scattered on the brain surface.
(This was obtained in collaboration with Drs. Akira Hashizume, Koji Iida and Kaoru Kurisu from the Department of Medicine at Hiroshima University.)
Three dimensional in-vivo imaging of electrical properties inside human bodies using magnetic resonance imaging (MRI)
It is known that malignant tissues have extraordinarily different electrical conductivity
and permittivity compared to normal tissues. Hence, three-dimensional, in-vivo imaging
of the electrical properties inside the human body has attracted attention for improving
cancer diagnosis techniques. Because conventional methods require an assumption that
the electrical properties do not spatially vary rapidly, the estimation results include
significant errors at the boundary between normal and malignant tissues, which is a
main region of interest in medical imaging. In our developed method, the electric field
is first identified from the measured magnetic field, and then the impedance is
reconstructed from this information. This direct reconstruction method is not only
theoretically simple and powerful, but also has an advantage in that the electrical
properties can be reconstructed at any arbitrary point inside the human body, even when
the electrical properties are spatially changing rapidly.
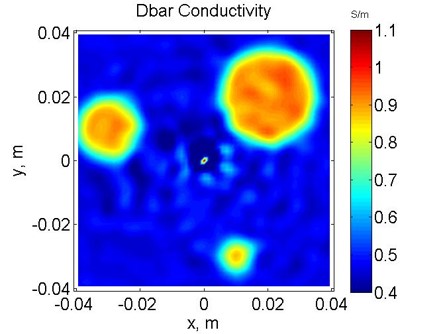
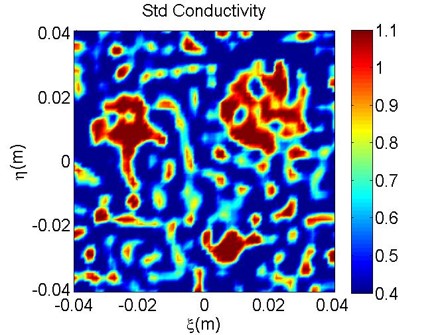
(left) Reconstructed conductivity using the proposed method and
(right) results using the conventional method.
This research is explained in the following invited lectures.
・
Industrial Applications of Complex Analysis
(Isaac Newton Institute, Univ. Cambridge): Mainly two-dimensional problems.
・
Inverse Problems and Nonlinearity 2021
(Helsinki, online conference): Mainly 3D problems.
Searching for victims buried in rubble
After an earthquake, searching for victims buried or trapped under rubble is a critically
important step in disaster response. We have developed a system that aims to locate
these individuals using their smartphones. To estimate the positions of smartphones,
the global positioning system (GPS) or the electromagnetic waves of WiFi are usually used
outdoors and indoors, respectively. However, when smartphones are surrounded by metallic objects,
these methods, which use high-frequency electromagnetic fields,
cannot estimate the positions accurately due to the reflection of the waves.
To circumvent this problem, we use a low-frequency magnetic field that is less affected
by surrounding metals and water. In practice, a rescuer generates a magnetic field using
a rotating magnetic dipole, which embeds the location information seen from the rescuer
in a space where victims may be buried. Then, the smartphones that the victims have measure the magnetic field,
compute their own locations, and send the information back to the rescuer. This method can
also be used for indoor navigation for humans or robots, which is an important aspect of cyber-physical systems.

See the following paper for more details:
A. Chiba and T. Nara, Three-dimensional localization of a rotating magnetic dipole from the Fourier integrals of its magnetic flux density with acceleration data, AIP Advances, vol. 10, no. 2, 025020, 2020.
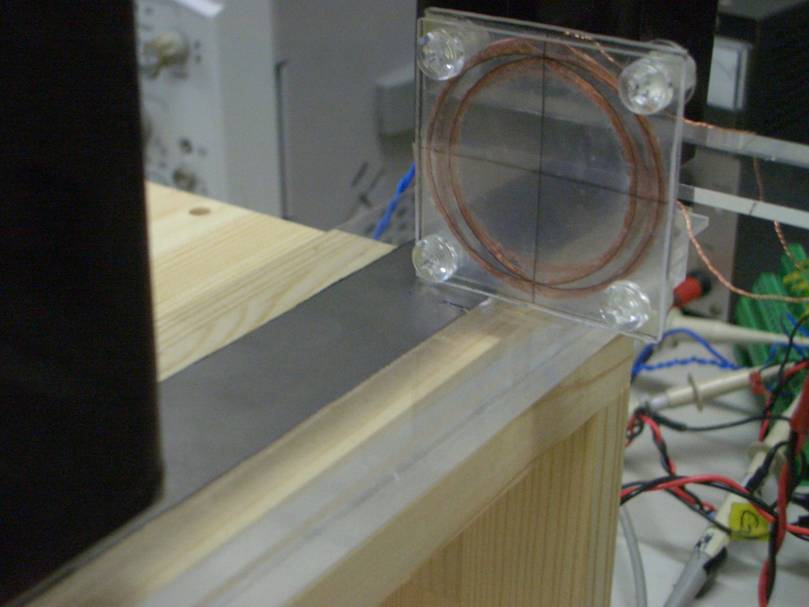
A sensor measuring Fourier coefficients for non-destructive inspection of ferromagnetic pipes
Automatic, accurate, rapid, and nondestructive inspection of infrastructures and power
plants is highly demanded. Among many methods using ultrasound waves and
electromagnetic fields, magnetic flux leakage methods are usually used for crack
detection in ferromagnetic materials. When magnetizing ferromagnetic plates or pipes
that have cracks, the magnetic flux leaks from the positions of the cracks. This magnetic
flux can then be measured and used to identify where the defects are.
Conventional methods use a magnetic sensor array composed of coils or Hall sensors,
and identify the crack position from the magnetic field distribution; these inspection
systems tend to be bulky and inconvenient to use. Another approach exists wherein a
single sensor is used to scan the whole structure; however, this has a disadvantage in
that it takes long time to complete the inspection.
In our laboratory, modeling a crack as a magnetic dipole, an explicit reconstruction
formula has been derived which estimates the crack position from the Fourier
coefficient of the leaked magnetic flux density. In addition, we have developed sensors
that can directly measure the Fourier cosine and sine coefficients of the magnetic field.

Using these two sensors only, the crack position in the pipe can be identified.
Overturning the common sense method of computing the Fourier coefficient/transform
from the weighted sum of sensor array outputs, we are now studying a structure capable
of measuring the integral transformation directly.
See the following papers for more details:
T. Nara, Y. Takanashi, and M. Mizuide, A sensor measuring the Fourier coefficients of the magnetic flux density for pipe crack detection using the magnetic flux leakage method, Journal of Applied Physics, vol. 109, 07E305, 2011.
T. Nara, M. Fujieda, and Y. Gotoh, Non-destructive inspection of ferromagnetic pipes based on the discrete Fourier coefficients of magnetic flux leakage, Journal of Applied Physics, vol. 115, 17E509, 2014.
Localization of RFID tags
Radio Frequency IDentification (RFID) tags are key elements in the Internet of Things (IoT).
In addition to a tag’s ID information, if its position is known, indoor navigation
applications or location-aware interfaces can be realized. We have developed algorithms
and sensors for locating low-frequency (135 kHz) RFID tags.
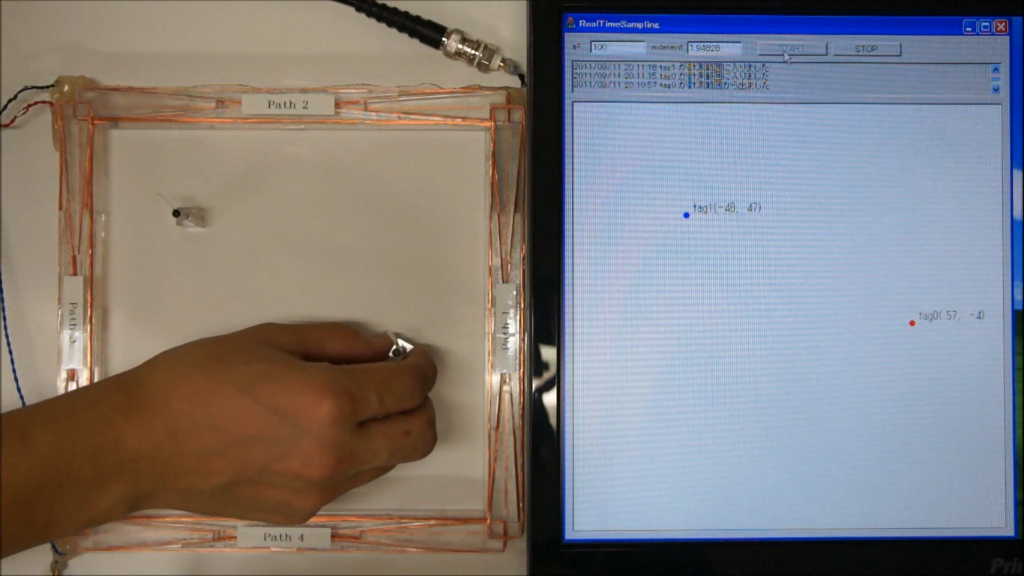
RFID tags located on a desk: when objects with RFID tags are repositioned on a desk,
their locations are estimated using three coils wound along the edges of the desk and are
shown on the screen with their ID numbers. A plural number of RFID tags can be also
identified by time division. The positional accuracy is about 5 mm within a 200 mm by
200 mm region. This system can be applied to, for example, safekeeping of medical bottles or medical prescriptions.
See the following paper for more details:
A. Chiba and T. Nara, 2-D Localization of Radio Frequency Identification tags from measurements of the weighted integrals of the magnetic flux density, IEEE Transactions on Magnetics, vol. 50, no. 9, 6200808, 2014.
Tactile display
Tactile displays are devices that can artificially provide a human with the sensation of
touching different textures. As visual and audio displays reproduce remote scenery
imaged by cameras and sound recorded by microphones, these devices can provide the
tactile sensation of touching the surfaces of remote objects. We have developed a
display using 10-MHz surface acoustic waves that control the friction between the
display and the surface of the skin, so that the sensation of roughness or smoothness can
be freely and continuously varied. Also, in order to effectively generate tactile
sensations, we have proposed mathematical models of the mechanoreceptors underneath
the skin that convert mechanical distortions to nerve signals.

See the following paper for more details:
T. Nara, M. Takasaki, T. Maeda, T. Higuchi, S. Ando, and S. Tachi, Surface Acoustic Wave Tactile Display, IEEE Computer Graphics and Applications, Vol. 21, No. 6, pp. 56-63, 2001.